
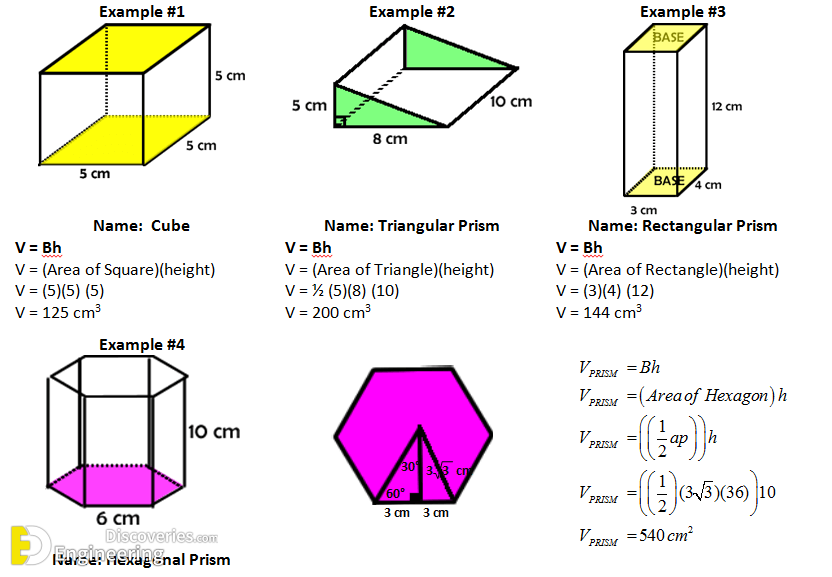
The formula for the volume of a hexagonal prism is, volume = a 2h cubic units where a is the base length and h is the height of the prism. What Is the Formula To Find the Volume of Hexagonal Prism? It is the product of the base area and the height of the prism. The volume of the hexagonal prism refers to the capacity of the hexagonal prism. Listed below are a few interesting topics related to the volume of hexagonal prism, take a look.įAQs on Volume of Hexagonal Prism What Is the Volume of Hexagonal Prism? Thus, the volume of the hexagonal prism is 332.544 cubic feet. Step 3: The volume of the hexagonal prism = base area × height = 332.544 × 8 = 332.544cubic feet.Step 2: The height of the prism is 8 ft.Step 1: The area of the base of the hexagonal prism is found using the formula, a 2 = (4) 2= 41.568 square feet.The steps to determine the volume of the hexagonal prism are: The volume of the hexagonal prism is obtained using the formula V =base area × height or a 2h. Solution: Given that base edge, a = 4 feet and height, h = 8 feet. Now refer to the example given below for more clarity.Įxample: Calculate the volume of the hexagonal prism with a base edge of 4 feet and a height of 8 feet.

#Volume of right prism calculator how to#
How To Calculate the Volume of Hexagonal Prism? An irregular hexagonal prism is a prism where all the sides of a hexagonal base do not have the same lengths.The angles of the regular hexagonal prism are also the same. A regular hexagonal prism is a prism with bases shaped like a hexagon with all the sides of the same length.regular hexagonal prisms and irregular hexagonal prisms. There are 2 different types of hexagonal prisms i.e. We can also use the formula V = 3abh, where Thus, the formula for the volume of a hexagonal prism is: Volume =area of base × height = a 2h cubic units where

The area of a regular hexagon with base length a is a 2 and height is h. As per the general formula of the volume of a prism, that is, volume = area of base × height, the formula for the volume of hexagonal prism = area of the hexagonal face x-height of the prism. The volume of a hexagonal prism determines the capacity of the prism. Thus, the volume of a hexagonal prism = area of base × height. By applying the above formula to a hexagonal prism. We know that the base of a hexagonal prism is a hexagon. We will use this formula to calculate the volume of a hexagonal prism as well. i.e., the volume of a prism = base area × height. The volume of any prism can be obtained by finding the product of its base area and its height. Then use it to estimate the volume lost to one indentation and multiply it by their number to get the actual chocolate filled volume.We will see the formulas to calculate the volumes of different types of hexagonal prisms. One way to approach this curious problem is to first use the volume of a prism calculator above to calculate the volume of the bar, including the indentations. Many camping tents are also such prisms, making use of the same beneficial properties.Ī triangular prism volume calculation may also be handy if you want to estimate the volume of a toblerone bar. This type of roof has the best distribution of forces generated by the weight of the roofing and lateral forces (i.e. Practical applicationsĪ lot of classical roofs have the shape of a triangular prism, so calculating the volume of air below it might be useful if you are using the space as a living area. For example, if the height is 5 inches, the base 2 inches and the length 10 inches, what is the prism volume? To get the answer, multiply 5 x 2 x 10 and divide the result by 2, getting 10 x 10 / 2 = 100 / 2 = 50 cubic inches. Three measurements of a prism need to be known before the volume can be calculated using the equation above: the prism length, height, and base.


 0 kommentar(er)
0 kommentar(er)
